Math Hombre

I love how Twitter works some days. OK, most days. #MTBoSfanboy

I retweet Scott's video talking about multiplication with his daughter. (He has lots of nice videos along these lines.)

 Martin responds with a connection.
 Brad shares the video.


 I respond, prompting a (as usually, intriguing) Dan tweet. Which will double as the moral of this blogpost.

Which Marilyn responds to... (talk about fanboy). And we will be looking into this trick.

Cool stuff in Nicholas' trick shared by Ms. Burns.

Links: Scott's post with his video, Brad's video, Marilyn's blog with her trick.

Since this wound up here, I thought I'd share how I subtract.

When I was in 2nd grade, Mrs. Schultz told us you can check subtraction by adding the result back. 6 year old mathchico thinks that if this is always correct, why don't we do that in the first place?

132 - 49? 3 plus 9 is 12 so 1+4 (now I'd say ten + forty) is 5, and 8 plus 5 is 13. Done, 83.

Over the years I've gotten in a lot of trouble for not borrowing.

One more:
 6+5=11.
 8+(6+1)=15
 7+(4+1)=12
 2+1=3.

Solved and checked, Mrs. Schultz!

Peace be to any teacher who had me in class, up to and including my advisor, Nigel Higson.






And Dan writes my signoff:

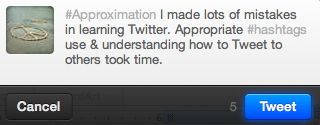
I had one of those great twitter moments yesterday, completely by generosity of tweeps. This captures one aspect of why I introduce twitter to our preservice teachers in hopes that they will either enter in or give it a go later.

My summer intermediate algebra class is leisurely (12 weeks instead of 6) picking its way through the summer. Linear, quadratics, exponentials with tons of technology use, simulation and experiment and a dash of art. And then comes logarithms.

My emphasis on introduction is as a way to undo exponentiation. Not a big emphasis on inverse functions, because though we're using the language, we haven't really dove into function language yet. But doing and undoing we talk about a lot.

But after that reasonably good start, we come up against the log rules. Our introduction was Kate Nowak's log law introduction (which I found through Sam Shah's Virtual Filing Cabinet; why bookmark? Let Sam do it for you.) The idea is that you see lots of examples and then try to generalize into a pattern, which is the log law. Nice pedagogy!

Yesterdays lesson included connecting the exponent rules to the log laws.





Tough going.  In terms of gradual release, we had to back up to a lot of teacher support. But the most useful law was the toughest. So I came back from class and just tweeted, to ... vent, I guess. Commiserate. (Which is definitely a purpose of twitter.)

 Wasn't expecting any real response. But then, the great feedback and constructive suggestions began immediately.

This is where we should start. We - as a teaching culture - are so steeped in general then specific, abstract before concrete, that this is a good first check.

 They did understand the multiplication to addition rule better. Look for areas where students understand and build off of those. Connections strengthen learning.

 This is the connection I'm going to follow up on. Look for a way to get it across.


 Think about the broader context. How the logarithm lives as an inverse function, with a nice concrete place to start.


 Support that this is challenging, and not me just being stupid. Of course, I'm happy when people point out I'm just being stupid, too, since I don't want to be stupid.

Neat post.  It gives a lot of good thinking about introducing logs, being intentional, and paying attention to students making sense of notation. This is very close to how I introduce logs, and how it looks like Kate Nowak introduces them, too. I use to think this was inappropriate for high school but okay for college (because of their bad early experiences), but I'm beginning to think that's how we should handle bad math notation. Use constructive notation and then transition the students to traditional.

 I don't think this is accessible (YET), but I'm going to mention this, too. Part of the class is getting the students more comfortable with symbolic methods, and I like how this emphasizes the operational part of logarithms.

A reminder of where to start. This is the fundamental relationship for logarithms, and connecting back to it well and often is important for learning in both directions.

So now I have a place to go Monday, following this up as I promised the students.  Maybe I would have had some of these insights on my own - but I don't have to work and think alone about my teaching or the mathematics. It also really sparked some thinking to me about whether logs should be introduced as a function or an operation. I feel like exponentiation goes from being notation, to an operation, to a function. Logarithms could do the same. I'm thinking:

Anyway, excellent teaching advice. I am so glad to be connected to these excellent teachers. And even though I can't go to Twitter Math Camp, I still get to have the home game to play. Why don't you play along?

(Here's my brief intro to twitter for math teachers. ) Here's the activity I put together for the next class:

From Rainbowcatz @ Flickr

I was gathering Standards Based Grading (SBG) resources for a colleague and thought that would be worth sharing.  Maybe this should be a LiveBinder? There's a definite math focus to my selections below, those it's not strict. Many people refer to SBG as Standards Based Assessment and Reporting (SBAR), which gets the whole 'grade' idea right out.

People: (Name links to Twitter)

• Jason Buell - SBG resources that includes the SBG galas.
• Shawn Cornally - collected resources at his blog
• Riley Lark - SBG blogposts
• Frank Noschese - SBG blogposts

Fundamentals and Further:

• Ed Leadership article: Seven Reasons for Standards-Based Grading by Patricia Scriffiny
• SBG Beginners Wiki, which also has math skills lists , started by Elissa Miller
• SBG page at the MSmathwiki.
• Blue Harvest - Shawn's SBG software
• ActiveGrade - Riley's SBG software

Twitter discussion

• #sbar - find more SBG folk, or people trying it in your discipline, by a Twitter search.
• #sbarbook - book group that 'meets' weekly for discussion about a particular book on assessment. Doesn't look like this semester's book is very engaging, though.

For completeness sake, here's my 2 (so far) SBG posts. Hey, this makes 3! If there are more examples of SBG in college, especially college math, please help me find them.

I missed a good #mathchat last night.  Chats are regularly scheduled twitter on-topic conversations. (Completish list; dizzying array of topics; mathchat is Thursday 8 pm (US-east) with a follow up on the same topic Monday at 3:30 pm.) You use twitters nice hashtag search feature to interact with people you may not typically follow (though it is a great place to find new follows).  Mathchat, started by Colin Graham (@colintgraham), has a wiki page where topics are voted on and chats are archived.  My current favorite way to follow is using TweetChat.

So if I missed the chat, how did I know it was a good one? There's the wiki archive, but also this time Bon Crowder (@mathfour) used Evernote to record and share the TweetChat record. Slick. It was good enough that I wanted to organize the list, which led me to figure to Tumbl it, but it turned into a blogpost when I realized the extent of the chat and that I had never blogged about mathchat before.

One thing that does not come across in this reorganized list is the conversational aspect. In Twitter you can click on a tweet and see all the previous tweets in that specific conversation.

People

• lostinrecursion must change misconception: you're either an applied person or a pure math person. Bleh
• mathheadinc  Misconception: Students below 10th grade can't be in college algebra, calculus...
• daveinstpaul Misconception: People with "math brains" learn math effortlessly.
• LinaSouid misconception: Math teachers only know/care/love math.
• LinaSouid misconception: Girls aren't good at math. Girls don't make good engineers.
• lostinrecursion must change misconception: it's ok to say "I'm so dumb at math. I'm not just not a math person." especially for kids
• delta_dc misconception: "I'm not good at math." form many of my preservice elementary teachers.
• LinaSouid A majority of elem teachers at my school hated math. Spread it to all their students like a disease.
• daveinstpaul If I have to pick just one misconception, it has to be "I'm not good at math."
• MrHonner For me, this S misconception: "I'm good at math, so it's not important to work hard to become a better writer."

Teaching

• lostinrecursion must change misconception: math is math class and homework should look like homework 
• lostinrecursion must change misconception: you really need to know algebra for the real world.
• lostinrecursion must change misconception: practice is the best way to get better. (personal experience actually is) 
• LinaSouid #mathchat misconception: Math teachers know how to solve every problem, ever!
• lostinrecursion must change misconception: you have to play the role of "teacher" to get class to work.
• TenMarks Misconception: Memorization is the only way to learn math. (I'm looking at you, multiplication tables!)
• lostinrecursion must change misconception: "they" write the problems. I just answer them. Can we tell what "they're" asking?
• @lostinrecursion must change misconception: "there are problems I need to solve. I need someone to show me how to do that." - Salman Khan!
• @lostinrecursion #mathchat must realize: math is made by humans like the ones in the classroom. So let's make math.  
•  lostinrecursion must change misconception: stick to the book or you're in trouble (aka I don't trust you)
• delta_dc Many of the misconceptions in math are result of learners trying to make sense w/o understanding. @graceachen has 2 nice posts.
• LinaSouid misconception:" With a graphing calculator I can do anything"--especially from over confident high school students.
• lostinrecursion must change misconception: you need the teacher to learn math. You need school to learn math.
• lostinrecursion must change misconception: texting in class is bad. (I'm in field theory class enjoying this much more) 
• rvdemerchant misconception: there is an old math and a new math.
• rvdemerchant old/new math? Did the old math think concept. understanding was bad? New math think procedural understanding bad? Nope
• delta_dc misconception: memorizing multiplication table will make one better at math. A lot of parents of MS kids say this.
• MrHonner I think the primary teaching misconception is "There is 1 right way to solve this problem."
• cheesemonkeysf IMHO this is also the primary TESTING misconception!
• MrHonner It is related to this misconception: "It is wrong for the teacher to admit they don't know something". 
• LinaSouid  I don't know when math teachers (20 years ago) learned that part of their job was to be socially inept
• mathheadinc Probably when they themselves were humiliated, probably at an early age. Vicious cycle

Field

• ColinTGraham For me, if it was only one chance... I would want to change the idea that mathematics is about doing calculations.
• MariaDroujkova Clear one misconceptions! math=arithmetic
• ekendriss Misconception I'd like to clear: Statistics = boring.
• MrHonner Major Math Misconception: There's only one right way to solve this problem. ColinTGraham Or: there *is* a right way....
• msnorthrup Luckily this is fading with elem tchrs RT @MrHonner the primary tchg misconception is "There's one right way to solve a problem"
• MrHonner Misconception: The most important thing is math is whether your answer is right or wrong
• MrHonner Student Misconception: "If I try something and it didn't work, I've done something wrong."
• shawn_ny That it lacks creativity.
• delta_dc Misconception - being fast at math means being good at math.
• LinaSouid misconception: You need to know how to prove something to use it and understand it.
• LinaSouid: misconception: You can't guess.
• lostinrecursion must change misconception: go with your first instinct. Your second is wrong (aka don't trust yourself)
• delta_dc Misconception - math is only worthwhile if it applies to real world.
• OoeyGooeyLady That u can "like" it but still struggle at it. I didn't need it 2 be easy. Just wanted to have chance to try!!
• LinaSouid So true. People focus on perfection. Another misconception!

Specific topics

• daveinstpaul Misconceptions: y is always a function of x. 0^0 cannot be consistently defined. √4 has two values. Everyone should take algebra.
• daveinstpaul  4 has two square roots, which are denoted √4 and -√4. By itself √4 indicates the positive square root.
• ColinTGraham By convention = accepted academic 'whatever'
• lostinrecursion must change misconception: fractions are wrong if they're not reduced.
• mathheadinc Misconception: This is not how to write a fraction (1 1/2)/4
• daveinstpaul Oh, another misconception: All polygons are convex.
• MathMatters2Me Misconception: radicals can never be left in the denominator
• MathMatters2Me Misconception: Asymptotes are lines a graph approaches but never touches
• MrHonner For purely math misconceptions, nothing beats sqrt(a^2 + b^2) = a + b.
• msnorthrup As a 5th grade teacher - the misconception that "You can't take a big number from a small number" is rampant and problematic
• daveinstpaul Misconception: When proving a trigonometric identity, you must work with one side of the equation at a time.
• MrCHRISatCSI You don't have to carry a one!

Is Google cooking the books?

Then what?

• LinaSouid Misconceptions will be resolved when math teachers are friendly, social, good role models
• ColinTGraham "friendly, social, good role models" depends very much on how people arrive at teaching mathematics...
• MariaDroujkova I am making a poster of today's #mathchat about misconceptions. So much concentrated math ed genius! You rock, #mathchat people!
• MrHonner We need to remind ALL teachers that they teach kids, not math. 

Think I'll have to at least lurk on Monday. I've been putting it on for background music during my preservice HS teacher class. (With occasional comments. Like you could resist.)

Photo credit: bfishshadow, petesimon @ Flickr

I had the good fortune to win a bet recently (well, best 2 out of 3) by the performance of the Yukon Huskies (jk) in the 2011 NCAA Division I men's Basketball Tournament.  My prize? A guestpost from Dave Coffey, @delta_dc.  (I was actually rooting for Butler, but that's a quality consolation prize!) This is Dave with Juneau.  Juneau is asking, "How could you bet on bulldogs?  Have I taught you nothing?  Haw!"

A few weeks back, one of our teacher assistants said, “You just started on Twitter this semester. I never would have guessed.” I wasn’t sure if she was talking about my quantity or quality. I chose quality and explained that it could be traced to Cambourne’s Conditions of Learning (a Foundational Framework of our Teacher Assisting Seminar).

This reminded me that John, my co-teacher, had asked me about blogging about the Conditions. I turned to him and said, “I’m thinking about writing about how the Conditions of Learning helped me to communicate using Twitter.” I thought this would be a good example of authentic learning in action.

The teacher assistant chimed in, “Maybe you could describe each condition in a Tweet.” John laughed, understanding that she had issued me a challenge without knowing it. Well, “challenge” accepted…


[Note from John - t was very tempting to put this in twitter-typical reverse order... but that would make it less readable.  Please forgive the lack of verisimilitude.]







Thanks, Dave and Jim Calhoun! And Brian Cambourne, of course.  The Reading Teacher has put the article introducing the Conditions online for download.  Or you can read the whole story in his book The Whole Story.  Also, I put the date on the cartoon at '95, the date of the RT article, but 1988 would be more accurate.

Photo Credit: Kathy Coffey, Rosaura Ochoa @ Flickr