Bendermeer Primary School Seminar for Parents
1 like854 views
This document provides an overview of a presentation on helping children with primary mathematics. It discusses how mathematics can develop intellectual competence and reflects on shifts in test questions to require more conceptual understanding and real-world problem solving over rote algorithms. Examples of math questions and lessons from various primary grades in Singapore, the US, UK, Netherlands and Japan are presented, covering topics like number sense, patterns, problem solving and visual models. Key competencies and strategies for problem solving are discussed.
1 of 41


































Ad
Recommended
Lower Primary Maths Problem Solving



Lower Primary Maths Problem SolvingJimmy Keng The document discusses various problem solving heuristics for lower primary mathematics. It provides 14 examples of word problems demonstrating different heuristics students can use to solve problems, such as using tables, looking for patterns, guess and check, and breaking problems into steps. The heuristics allow students to systematically solve problems even if they are initially unfamiliar.
Parents Seminar on Heuristics at Chongfu School



Parents Seminar on Heuristics at Chongfu SchoolJimmy Keng This is the set of slides used at the seminar for Primary Five parents at Chongfu School in March 2011.
2010 PSLE Mathematics Seminar for Parents



2010 PSLE Mathematics Seminar for ParentsJimmy Keng Seminar for Parents
Fairfield Methodist Primary School & Griffiths Primary School
26 & 27 February 2010
How to Ace PSLE Maths



How to Ace PSLE MathsJimmy Keng The document provides information about preparing for and taking the PSLE Mathematics exam in Singapore. It discusses the structure of the exam, which consists of two papers, and outlines the curriculum focus on problem solving. It also provides examples of different types of math problems students may encounter on the exam. At the end, it discusses a news article where parents complained that this year's PSLE math exam was unusually difficult, possibly because it was the first year calculators were allowed.
Multiplication & division teaching ideas



Multiplication & division teaching ideasJoanne Villis This document provides 16 teaching ideas for teaching multiplication and division to students. The teaching ideas include revising number patterns online, investigating multiples, using visual representations and words to teach concepts, creating instructional videos and songs with QR codes, using apps and games to practice, exploring arrays with blocks and in the environment, playing games like the array game to practice, creating a multiplication pyramid together, and using strategies like Study Ladder for rapid recall practice. Bloom's Taxonomy and Multiple Intelligences are also incorporated into activity ideas.
Singapore math @ sheraton



Singapore math @ sheratonguest009ebb The document discusses the theoretical underpinnings of the Singapore Math approach. It describes three key theories that influenced the development of Singapore Math:
1. Bruner's theory of the concrete-pictorial-abstract approach to help students develop a strong mathematical foundation.
2. Skemp's theory of conceptual understanding versus procedural understanding, with Singapore Math emphasizing the former.
3. Dienes' theory of variation in mathematics education through perceptual and mathematical variability in tasks.
The document provides examples of how these theories are applied in Singapore Math teaching materials, lessons, and instructional models.
Contoh strategi penyelesaian masalah dalam polya’s model



Contoh strategi penyelesaian masalah dalam polya’s modelenseriban The document describes strategies for solving word problems using Polya's model of problem solving. It provides examples of applying different strategies - finding a pattern, making a table, working backwards, guessing and checking, drawing a picture, making a list, and writing a number sentence. Each strategy example follows the four-step Polya model of understanding the problem, planning a solution, carrying out the plan, and checking the solution.
CLASS V MATHS DIVISION



CLASS V MATHS DIVISIONRc Os This document discusses two types of division problems: equal sharing and grouping. Equal sharing involves dividing a quantity into equal portions to determine how much each portion contains. Grouping involves determining how many portions of a given size can be obtained from a given total quantity. Examples are provided of dividing bananas equally among children and packing sweets into packets of a set number.
joyful_learning_of_mathematics



joyful_learning_of_mathematicsArchana Dwivedi This document discusses various tools and techniques for teaching mathematics creatively and joyfully. It emphasizes the need to use blended strategies to engage different types of learners and develop higher-order thinking skills. Some recommended approaches include using different types of papers, foldables, games like sudoku and puzzles, interactive applets, collaborative projects, blogs, appreciating math in everyday examples, origami, peer teaching and more. The goal is to make math accessible and encourage passion for learning through independent and creative environments.
meanings of division



meanings of divisionAlex Blank This document provides instruction on teaching students the concept of division through modeling with connecting blocks and drawing representations. It explains that division can be thought of as repeated subtraction or forming equal groups. Students will practice writing division problems based on word problems by modeling with connecting blocks and writing the problems with the dividend, divisor, and quotient. If they finish early, they can make up their own division word problems for their partner.
Interim 1 Review



Interim 1 ReviewLauren Pressley The document contains multiple math word problems and questions. It asks the reader to evaluate expressions, find missing values, draw graphs, choose the student with the correct solution, write equations, and determine which variable had the least variation from data. It also provides tables with numbers and asks questions about the data.
Mahasarakham Rajabhat University Day 1



Mahasarakham Rajabhat University Day 1Jimmy Keng Rajabhat Mahasarakham organised this workshop titled Transforming the Mathematics Classroom. The goal is to get teachers to think about teaching mathematics to encourage thinking, to develop visualization and to enhance the ability to observe patterns rather than mathematics as a subject that requires memorization, carrying out meaningless procedures and doing tedious computations.
Translations of real-verbal expressions into letters or symbols and vice versa.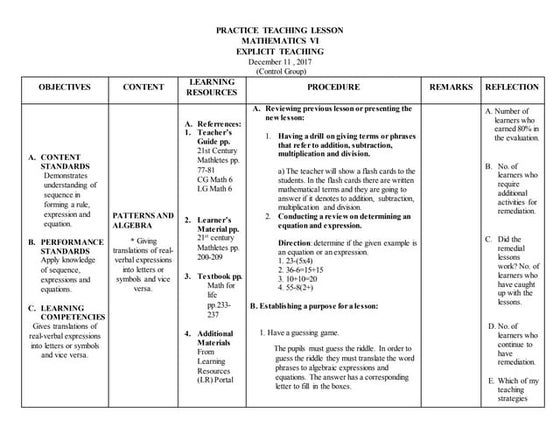



Translations of real-verbal expressions into letters or symbols and vice versa.April Rose Anin Giving translations of real-verbal expressions into letters or symbols and vice versa an explicit lesson Plan for the K-12 Curriculum
8 step model drawing



8 step model drawingSitiIshak7 This document provides an overview of the 8-step model drawing strategy used in Singapore Math to help students solve word problems. It explains that model drawing is a key strategy that uses bars or diagrams to represent quantities in word problems visually. It then demonstrates how to set up and solve a variety of word problems using the 8-step model drawing approach through worked examples. The examples cover topics like addition, subtraction, fractions, ratios, percentages and more.
Singapore Math Administrators Symposium, Chicago 



Singapore Math Administrators Symposium, Chicago Jimmy Keng This national edition of the symposium was held in Chicago. This was Dr Yeap Ban Har's day-long presentation. Dr Duriya Aziz, Andy Clark, Dr Richard Bisk and Dr Steve Leinwand were among the other presenters.
Number sentences j villis



Number sentences j villisJoanne Villis This slide share has higher order thinking ways of teaching students to understand the relationship between the four number operations. This process have been a trial and error process for me, I have loved working with students along the way. Online and iPad resources have been provided.
Problem Solving



Problem SolvingSyaza Yasmin Polya's four step process for problem solving includes: 1) understanding the problem fully, 2) devising a plan or strategy to solve it, 3) carrying out the plan, and 4) looking back at the solution critically. Some alternative methods for solving non-routine problems discussed applying related problems, simplifying or restating the problem, working backwards, and considering auxiliary elements.
Masterclass Utrecht MAP101



Masterclass Utrecht MAP101Jimmy Keng This document summarizes a presentation on Singapore Math. It discusses how Singapore Math focuses on thinking, visualization using bar models, patterns and generalization, problem solving, and applying knowledge through problem solving. It provides examples of how concepts like fractions division and place value are taught using hands-on approaches like paper folding rather than solely drill-and-practice.
Multiplication and division



Multiplication and divisionKhairani Rani The document explains how multiplication and division are related. Multiplication is a shortcut for addition of equal groups, while division is the opposite of multiplication and involves splitting things into equal groups. Examples are provided to illustrate how to use multiplication to solve division problems by thinking of the division sign as asking "what number multiplied by the given number equals the total?"
Teaching Fractions using Visuals



Teaching Fractions using VisualsJimmy Keng This document discusses using visual representations to teach fractions, including concrete objects like fraction discs and pictorial models. It recommends first building conceptual understanding before teaching procedural skills like computation. Key concepts covered include part-whole relationships, mixed numbers and improper fractions, multiplication and division of fractions using concrete examples, and word problems.
Sequencing (Formulating the nth term rule) Explicit lesson plan grade VI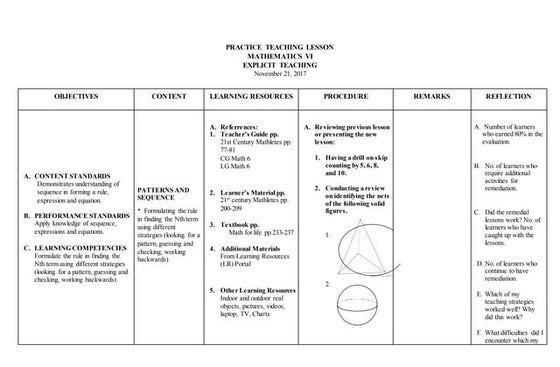



Sequencing (Formulating the nth term rule) Explicit lesson plan grade VIApril Rose Anin This was just my lesson plan during my practice teaching. Hope this will serve as a reference to all of you.
Multiplication keynote presentation



Multiplication keynote presentationPhoebe Peng-Nolte The document provides instructions for multiplying by 9 using patterns. It shows that the tens digit of the product is 1 less than the number being multiplied by 9. The ones digit is the difference between 9 and the tens digit. It then asks the reader to use this pattern to find the product of 6 x 9, which is 54.
AME-SMS Conference 2012 Secondary



AME-SMS Conference 2012 SecondaryJimmy Keng This lecture discusses strategies for incorporating reflection in mathematics classrooms. It provides examples of reflection techniques that can be used in both teacher-centered lessons like lectures as well as student-centered lessons like group work. Some strategies mentioned include using board writing, math journals, student explanations, self-assessments, problem-solving approaches, and questioning techniques to encourage students to re-examine their thinking. The goal is to promote deep learning through reflection on mathematical concepts and ideas.
Henry Park PSLE Seminar for Parents



Henry Park PSLE Seminar for ParentsJimmy Keng This seminar for parents discussed the five key competencies in mathematical problem solving. Parental involvement is an important aspect of helping kids do well in school.
AMSTI Event, Birmingham, Alabama



AMSTI Event, Birmingham, AlabamaJimmy Keng This document summarizes Singapore's approach to mathematics education. It discusses how Singapore Math emphasizes conceptual understanding through concrete experiences and visual representations. It highlights Singapore's high performance on international assessments like TIMSS and PISA. It also outlines recent developments in Singapore's mathematics curriculum, pedagogy, textbooks, and use of technology and learning support strategies to help all students succeed.
Story Problems



Story Problemspkarish The document provides examples and step-by-step solutions to mathematical word problems involving functions, equations, and algebraic modeling. It includes 8 sample word problems, showing the work to determine an appropriate equation or function to model each problem and solve to find the unknown values. The overall goal is to help students analyze problems, determine solution strategies using algebra, and evaluate whether solutions make sense in context.
Surviving Math! 3



Surviving Math! 3Jimmy Keng This document provides a summary of a presentation on surviving math given by Dr. Yeap Ban Har from the Marshall Cavendish Institute in Singapore. The presentation included slides available on Facebook and discussed shifts in math test questions over time towards requiring more conceptual understanding. It also showed sample math problems and performance data from Primary 4 students in Singapore on TIMSS tests. The document lists the speaker, ___location, contact information and source of additional slides.
First New Teachers' Conference, Manila, 10 September 2011



First New Teachers' Conference, Manila, 10 September 2011Jimmy Keng This document contains summaries from presentations given at various schools around the world on teaching mathematics. It discusses lessons from Chile, Japan, and the Philippines on public lessons, as well as a LEAP program in Manila. Participants at Keys Grade School in Manila provided methods for finding the difference between two numbers. At De Tweemaster in the Netherlands, participants demonstrated methods for finding the area of polygons by counting dots. The document outlines three main points about teaching mathematics through visualization, generalization, and communication. It provides further examples and solutions for percentage and angle problems.
Surviving math
Surviving mathJimmy Keng I do not actually have enough context to determine the categories that numbers would fall under. Numbers themselves do not inherently fall under letters or categories.
Ad
More Related Content
What's hot (18)
joyful_learning_of_mathematics



joyful_learning_of_mathematicsArchana Dwivedi This document discusses various tools and techniques for teaching mathematics creatively and joyfully. It emphasizes the need to use blended strategies to engage different types of learners and develop higher-order thinking skills. Some recommended approaches include using different types of papers, foldables, games like sudoku and puzzles, interactive applets, collaborative projects, blogs, appreciating math in everyday examples, origami, peer teaching and more. The goal is to make math accessible and encourage passion for learning through independent and creative environments.
meanings of division



meanings of divisionAlex Blank This document provides instruction on teaching students the concept of division through modeling with connecting blocks and drawing representations. It explains that division can be thought of as repeated subtraction or forming equal groups. Students will practice writing division problems based on word problems by modeling with connecting blocks and writing the problems with the dividend, divisor, and quotient. If they finish early, they can make up their own division word problems for their partner.
Interim 1 Review



Interim 1 ReviewLauren Pressley The document contains multiple math word problems and questions. It asks the reader to evaluate expressions, find missing values, draw graphs, choose the student with the correct solution, write equations, and determine which variable had the least variation from data. It also provides tables with numbers and asks questions about the data.
Mahasarakham Rajabhat University Day 1



Mahasarakham Rajabhat University Day 1Jimmy Keng Rajabhat Mahasarakham organised this workshop titled Transforming the Mathematics Classroom. The goal is to get teachers to think about teaching mathematics to encourage thinking, to develop visualization and to enhance the ability to observe patterns rather than mathematics as a subject that requires memorization, carrying out meaningless procedures and doing tedious computations.
Translations of real-verbal expressions into letters or symbols and vice versa.



Translations of real-verbal expressions into letters or symbols and vice versa.April Rose Anin Giving translations of real-verbal expressions into letters or symbols and vice versa an explicit lesson Plan for the K-12 Curriculum
8 step model drawing



8 step model drawingSitiIshak7 This document provides an overview of the 8-step model drawing strategy used in Singapore Math to help students solve word problems. It explains that model drawing is a key strategy that uses bars or diagrams to represent quantities in word problems visually. It then demonstrates how to set up and solve a variety of word problems using the 8-step model drawing approach through worked examples. The examples cover topics like addition, subtraction, fractions, ratios, percentages and more.
Singapore Math Administrators Symposium, Chicago 



Singapore Math Administrators Symposium, Chicago Jimmy Keng This national edition of the symposium was held in Chicago. This was Dr Yeap Ban Har's day-long presentation. Dr Duriya Aziz, Andy Clark, Dr Richard Bisk and Dr Steve Leinwand were among the other presenters.
Number sentences j villis



Number sentences j villisJoanne Villis This slide share has higher order thinking ways of teaching students to understand the relationship between the four number operations. This process have been a trial and error process for me, I have loved working with students along the way. Online and iPad resources have been provided.
Problem Solving



Problem SolvingSyaza Yasmin Polya's four step process for problem solving includes: 1) understanding the problem fully, 2) devising a plan or strategy to solve it, 3) carrying out the plan, and 4) looking back at the solution critically. Some alternative methods for solving non-routine problems discussed applying related problems, simplifying or restating the problem, working backwards, and considering auxiliary elements.
Masterclass Utrecht MAP101



Masterclass Utrecht MAP101Jimmy Keng This document summarizes a presentation on Singapore Math. It discusses how Singapore Math focuses on thinking, visualization using bar models, patterns and generalization, problem solving, and applying knowledge through problem solving. It provides examples of how concepts like fractions division and place value are taught using hands-on approaches like paper folding rather than solely drill-and-practice.
Multiplication and division



Multiplication and divisionKhairani Rani The document explains how multiplication and division are related. Multiplication is a shortcut for addition of equal groups, while division is the opposite of multiplication and involves splitting things into equal groups. Examples are provided to illustrate how to use multiplication to solve division problems by thinking of the division sign as asking "what number multiplied by the given number equals the total?"
Teaching Fractions using Visuals



Teaching Fractions using VisualsJimmy Keng This document discusses using visual representations to teach fractions, including concrete objects like fraction discs and pictorial models. It recommends first building conceptual understanding before teaching procedural skills like computation. Key concepts covered include part-whole relationships, mixed numbers and improper fractions, multiplication and division of fractions using concrete examples, and word problems.
Sequencing (Formulating the nth term rule) Explicit lesson plan grade VI



Sequencing (Formulating the nth term rule) Explicit lesson plan grade VIApril Rose Anin This was just my lesson plan during my practice teaching. Hope this will serve as a reference to all of you.
Multiplication keynote presentation



Multiplication keynote presentationPhoebe Peng-Nolte The document provides instructions for multiplying by 9 using patterns. It shows that the tens digit of the product is 1 less than the number being multiplied by 9. The ones digit is the difference between 9 and the tens digit. It then asks the reader to use this pattern to find the product of 6 x 9, which is 54.
AME-SMS Conference 2012 Secondary



AME-SMS Conference 2012 SecondaryJimmy Keng This lecture discusses strategies for incorporating reflection in mathematics classrooms. It provides examples of reflection techniques that can be used in both teacher-centered lessons like lectures as well as student-centered lessons like group work. Some strategies mentioned include using board writing, math journals, student explanations, self-assessments, problem-solving approaches, and questioning techniques to encourage students to re-examine their thinking. The goal is to promote deep learning through reflection on mathematical concepts and ideas.
Henry Park PSLE Seminar for Parents



Henry Park PSLE Seminar for ParentsJimmy Keng This seminar for parents discussed the five key competencies in mathematical problem solving. Parental involvement is an important aspect of helping kids do well in school.
AMSTI Event, Birmingham, Alabama



AMSTI Event, Birmingham, AlabamaJimmy Keng This document summarizes Singapore's approach to mathematics education. It discusses how Singapore Math emphasizes conceptual understanding through concrete experiences and visual representations. It highlights Singapore's high performance on international assessments like TIMSS and PISA. It also outlines recent developments in Singapore's mathematics curriculum, pedagogy, textbooks, and use of technology and learning support strategies to help all students succeed.
Story Problems



Story Problemspkarish The document provides examples and step-by-step solutions to mathematical word problems involving functions, equations, and algebraic modeling. It includes 8 sample word problems, showing the work to determine an appropriate equation or function to model each problem and solve to find the unknown values. The overall goal is to help students analyze problems, determine solution strategies using algebra, and evaluate whether solutions make sense in context.
Similar to Bendermeer Primary School Seminar for Parents (20)
Surviving Math! 3



Surviving Math! 3Jimmy Keng This document provides a summary of a presentation on surviving math given by Dr. Yeap Ban Har from the Marshall Cavendish Institute in Singapore. The presentation included slides available on Facebook and discussed shifts in math test questions over time towards requiring more conceptual understanding. It also showed sample math problems and performance data from Primary 4 students in Singapore on TIMSS tests. The document lists the speaker, ___location, contact information and source of additional slides.
First New Teachers' Conference, Manila, 10 September 2011



First New Teachers' Conference, Manila, 10 September 2011Jimmy Keng This document contains summaries from presentations given at various schools around the world on teaching mathematics. It discusses lessons from Chile, Japan, and the Philippines on public lessons, as well as a LEAP program in Manila. Participants at Keys Grade School in Manila provided methods for finding the difference between two numbers. At De Tweemaster in the Netherlands, participants demonstrated methods for finding the area of polygons by counting dots. The document outlines three main points about teaching mathematics through visualization, generalization, and communication. It provides further examples and solutions for percentage and angle problems.
Surviving math
Surviving mathJimmy Keng I do not actually have enough context to determine the categories that numbers would fall under. Numbers themselves do not inherently fall under letters or categories.
Red Swastika School



Red Swastika SchoolJimmy Keng The document discusses various problem-solving heuristics or methods that can be used in mathematics, including:
1) The bar model method which involves visualizing a problem using bars or diagrams to represent quantities.
2) Using guess and check or making a table to systematically try values in a problem.
3) Breaking problems into number sentences to write mathematical expressions that can then be solved.
4) Looking for patterns or structures in numbers that can provide clues for solving problems.
Numeracy Oct 23 -Denise Flick



Numeracy Oct 23 -Denise FlickSchool District #20 Today's agenda includes a math lesson covering personal strategies for addition, subtraction, multiplication, and division. The schedule also includes a nutrition break, looking at virtual manipulatives and resources, lunch, and an assessment period. The document discusses teaching math concepts conceptually rather than procedurally and the importance of understanding operations rather than just memorizing computations. It provides examples of story problems and strategies adults use to solve math problems informally in everyday life.
Story Problems



Story Problemspkarish The document provides examples and step-by-step solutions to mathematical word problems involving functions, equations, and algebraic modeling. It includes 8 sample word problems, showing the process of setting up and solving equations to determine unknown values. The overall goal is to help students analyze problems, determine appropriate solution strategies using algebraic notations, and evaluate solutions in context.
DLL_MATHEMATICS 5_Q2_W9.docx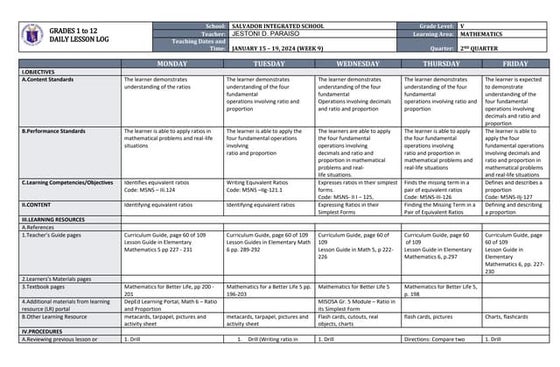



DLL_MATHEMATICS 5_Q2_W9.docxJestoniParaiso1 This document contains a daily lesson log for a 5th grade mathematics class. The objectives are for students to understand ratios, equivalent ratios, and the four fundamental operations involving ratios and proportions. On the days logged, the teacher planned to review identifying equivalent ratios, expressing ratios in simplest forms, and defining and describing proportions. Examples and activities were provided to practice these skills, including word problems, games, and group work. The goal was for students to apply ratio concepts to mathematical problems and real-world situations.
W2 MATH.pptx TIS IS ALL ABOUT POWER POINT OIN MY SUBJECT FOR WEEK 2



W2 MATH.pptx TIS IS ALL ABOUT POWER POINT OIN MY SUBJECT FOR WEEK 2LesliePulod Solve routine and non-routine problems involving factors, multiples, and divisibility rules for 2, 3, 4, 5, 6, 8, 9, 10, 11, and 12.
● Solving Problems on Divisibility Rules
⮚ Solves routine and non-routine problems involving factors, multiples, and divisibility rules for 2,3,4,5,6,8,9,10,11, and 12. (M5NS-Ic-59)
Ipil Elementary School is set to conduct a tree planting activity on September 1, 2020. All Grade 5 boys are expected to plant 50 seedlings, and the
Grade 5 girls are to plant 30 seedlings. They will plant the seedlings in such a way that the trees will grow in equal numbers per row. What is the possible common
number of trees in each row?
Ipil Elementary School is set to conduct a tree planting activity on September 1, 2020. All Grade 5 boys are expected to plant 50 seedlings, and the
Grade 5 girls are to plant 30 seedlings. They will plant the seedlings in such a way that the trees will grow in equal numbers per row. What is the possible common
number of trees in each row?
FIRST GRADING



FIRST GRADINGEunicePama This document provides lessons on mathematical concepts and operations involving fractions, including:
1) Multiplying fractions mentally with denominators up to 10 by multiplying the numerators and denominators.
2) Visualizing division of fractions using illustrations to represent the operations.
3) Using rules for divisibility to determine if a number is divisible by 3, 6, 9, 4, 8, or 12 in order to find common factors.
Chile MOE Seminar English



Chile MOE Seminar EnglishJimmy Keng This is the English version of the presentation at Avancemos en las Matematicas: La Mirada de Singapur
Association of Mathematics Educators Seminar on PSLE Mathematics



Association of Mathematics Educators Seminar on PSLE MathematicsJimmy Keng This seminar was for parents and teachers interested in helping students in preparing for the PSLE Mathematics.
Mentari Upper Primary Number Sense



Mentari Upper Primary Number SenseJimmy Keng This is the course or teachers in Indonesia on number sense for Primary 4 to 6. It covers place values, regrouping, large number multiplication and division and some ideas on estimation and multiples.
(7) Lesson 6.6



(7) Lesson 6.6wzuri The document provides examples for solving word problems using the work backward strategy. It includes three example problems and their answers:
1) Miguel had originally $27 based on spending amounts for food and ice cream and having $9 left which was 1/3 of the original.
2) Curtis' starting vet bill balance was $160 based on an $80 original bill, $40 added for medicine, and now owing $120.
3) The number is 21 based on dividing a number by 3, adding 5 to the quotient, and subtracting 2 to get 10.
Professional Development Session for Teachers in California by singaporemath....



Professional Development Session for Teachers in California by singaporemath....Jimmy Keng The document discusses the theoretical underpinnings of the Singapore Math approach. It describes three key theories that influenced the development of Singapore Math:
1. Bruner's theory of concrete-pictorial-abstract learning, which emphasizes using concrete objects before moving to pictorial then abstract representations.
2. Skemp's theory of instrumental versus relational understanding, which stresses the importance of conceptual understanding over rote learning.
3. Dienes' theory of variation, which promotes using varied examples and representations to enhance conceptual understanding.
The document explains how these theories influenced the instructional models, curriculum design, and assessment in Singapore Math to develop students' conceptual understanding and mathematical thinking.
Professional Development for Californian Teaching Teaching Singapore Math



Professional Development for Californian Teaching Teaching Singapore MathJimmy Keng This presentation was part of a one-day workshop for teachers in California who teach Singapore Math. It was organized by singaporemath.com
Math Plus Kansas City



Math Plus Kansas CityJimmy Keng The document discusses using math journals in elementary school classrooms to teach mathematical concepts. It describes a framework for teaching mastery that involves anchor tasks, guided practice, and independent practice. Journaling is presented as one part of this process where students explore concepts, structure their understanding, and reflect on their learning. Examples are provided of kindergarteners recording number bonds in their journals to master basic counting and addition. The journal entries allow teachers to assess understanding and provide differentiated instruction.
Outreach p6-math@



Outreach p6-math@Azis Ibrahim 1. The document is about a book titled "Primary 6 Mathematics Ace The Exams with My 24/7 Personal Tutor" which contains 10 mock exam papers and detailed explanations for Primary 6 math questions on a CD-ROM.
2. The book is meant to help pupils prepare for Primary 6 math exams by including exam-style questions that highlight common misconceptions.
3. The accompanying CD-ROM contains video lessons explaining how to solve each question in the mock exams.
Outreach p6-math.#



Outreach p6-math.#Azis Ibrahim 1. The document is about a book titled "Primary 6 Mathematics Ace The Exams with My 24/7 Personal Tutor" which contains 10 mock exam papers and detailed explanations for Primary 6 math questions on a CD-ROM.
2. The book is intended to help pupils prepare for Primary 6 math exams by including exam-style questions that highlight common misconceptions.
3. The accompanying CD-ROM contains video lessons explaining the solutions to each question in the mock exams.
Ad
More from Jimmy Keng (20)
Maths Hub June 2015 Day 1 and Day 2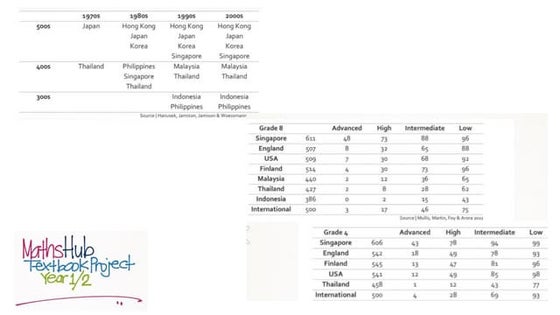



Maths Hub June 2015 Day 1 and Day 2Jimmy Keng This document discusses differentiating instruction to challenge advanced learners. It provides examples of ways to assess basic subtraction skills through runway indicators. For advanced learners, it suggests having them solve problems in alternative ways, write stories for equations, or write notes applying math concepts to enrich their learning beyond basic skills.
Primary Mathematics Fractions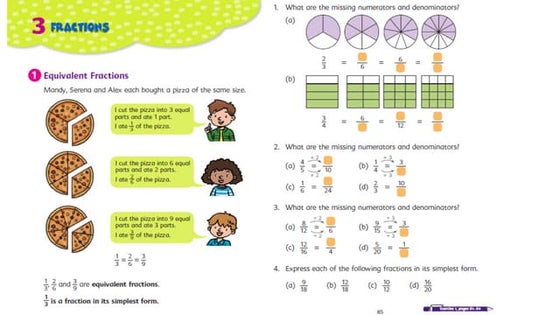



Primary Mathematics FractionsJimmy Keng Basic Lesson Structure of Singapore Math - anchor task, guided practice and independent practice
Blake School Presentation Grade 1



Blake School Presentation Grade 1Jimmy Keng The document outlines a schedule for four sessions occurring between 08:30-04:00 with a forum period from 03:30-04:00. It then discusses using a lesson on the Common Core to understand critical areas for Grade 1, including number bonds, comparison of numbers, and conservation of numbers. Various methods for developing visualization like the CPA approach, bar models, and teacher questioning are listed. The document goes on to discuss elements of effective math lessons including exploration, structure, journaling, reflection, and guided/independent practice using addition and subtraction strategies like counting all, making 10, subtracting from ten, and renaming before subtracting with examples provided.
Blake Grade 6



Blake Grade 6Jimmy Keng This document provides an overview of a middle school mathematics institute that will take place on Saturday. It discusses the basic lesson format, which includes an anchor task, guided practice, and independent practice. It also references Bruner's idea of using concrete experiences and pictorial representations to help students understand abstract ideas. The document then provides several case studies as examples of lessons that could be used to develop, apply, and practice various mathematical concepts involving fractions, algebra, geometry, and more.
Blake grade 5



Blake grade 5Jimmy Keng This document provides an overview of a Grade 5 mathematics institute that will take place on a Friday. It outlines the basic lesson format, which includes an anchor task, guided practice, and independent practice. It also references several learning theories that provide a framework for the lesson, such as Bruner's idea of concrete, iconic, and symbolic representations. The document includes several math word problems from past PSLE exams in Singapore to use for practice. It concludes with key learning theories covered and strategies for challenging advanced learners.
Blake Grade 4



Blake Grade 4Jimmy Keng 1) The document discusses standards and instructional focus areas for Grade 4 mathematics, including multi-digit multiplication and division, fractions, and geometric shapes.
2) It provides examples of case studies to solve, including arranging fractions in order and word problems involving fractions of amounts.
3) Theories of learning and representation discussed include Bruner's CPA approach and Skemp's classifications of understanding in mathematics.
Blake Grade 3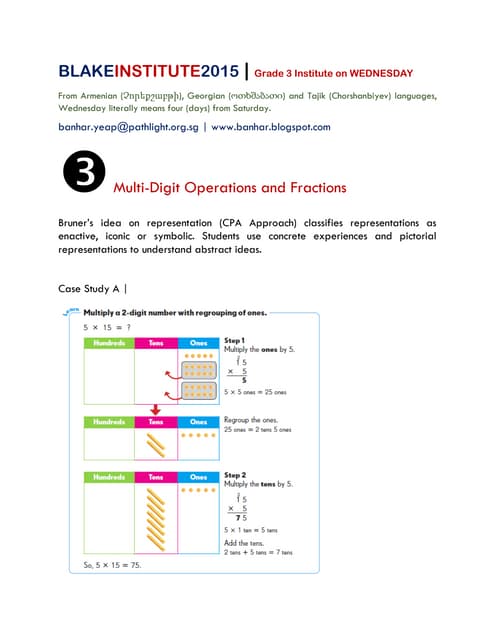



Blake Grade 3Jimmy Keng This document provides information about a grade 3 mathematics institute to be held on Wednesday. It discusses key theories in mathematics learning from Piaget, Bruner, Dienes, Vygotsky, and Skemp. The document also provides several case studies with examples of lesson plans and problems that assess different mathematical concepts like operations, fractions, problem solving, and place value.
Blake Grade 2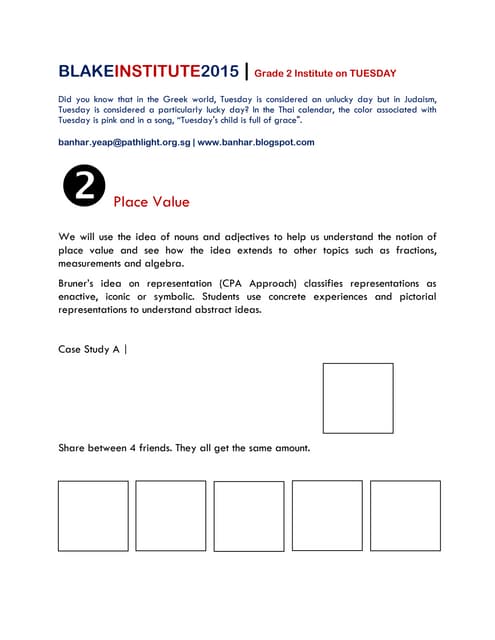



Blake Grade 2Jimmy Keng The document discusses a Grade 2 math institute that will focus on place value using concrete and pictorial representations. It provides several case studies on topics like subtraction across zeros, fractions, and problem solving. The lessons follow a basic format of an anchor task, guided practice, and independent practice. Key learning theories that will be drawn from include those from Piaget, Bruner, Dienes, Vygotsky, and Skemp. Bruner's CPA approach and Skemp's classifications of understanding will be particularly relevant for using different representations to build conceptual and relational understanding.
Blake Grade 1



Blake Grade 1Jimmy Keng Participants to Blake School Singapore Math Institute in June 2015 please download and print this set of handouts.
2104 WSU Cutouts



2104 WSU CutoutsJimmy Keng This document contains instructions for cutting out numbers and mathematical symbols from a page. It includes numbers from 0 to 9, basic mathematical operators like + and -, and instructions to cut out the listed items. The document is repetitive, listing the same numbers and symbols twice.
2014 WSU Handouts



2014 WSU HandoutsJimmy Keng The document summarizes discussions from breakout sessions at the 4th Singapore Math Institute on teaching mathematics using Singapore's approach. It provides tasks and problems from Singapore classrooms related to practicing skills through problem-solving, using anchor tasks to structure lessons, and teaching geometry in grades 4-6. The goals are to emulate Singapore's emphasis on problem-solving and multi-step word problems to develop students' mathematical thinking.
2014 WSU Day 2



2014 WSU Day 2Jimmy Keng The document discusses strategies for developing and improving mathematical practices, such as using anchor tasks, collaborative structures, questioning techniques, journals, textbooks for reflection, and focusing on visualization, generalization, and number sense. It also discusses moving from concrete to pictorial to abstract representations, and using anchor tasks and challenging word problems to teach Singapore math concepts.
2014 WSU Day 1 Slides



2014 WSU Day 1 SlidesJimmy Keng This document discusses using a problem-solving approach to teach mathematics. It focuses on practicing problem-solving lessons and breaking students into groups to work on problems. The document provides resources for lesson plans and contact information for the speaker who advocates for integrating problem-solving into mathematics education.
Se01 abc's of singapore math through whole numbers



Se01 abc's of singapore math through whole numbersJimmy Keng The document discusses the Singapore approach to teaching mathematics, which was developed to help Singaporean students perform better in math. It is based on Bruner's ideas of a spiral curriculum and using representations. The document provides 15 sample math tasks that demonstrate fundamentals of Singapore Math instruction, such as the Concrete-Pictorial-Abstract approach. It also includes quotes from Bruner about revisiting basic ideas repeatedly to help students master structured bodies of knowledge.
Sd09 visualization



Sd09 visualizationJimmy Keng This document contains an agenda for a staff development conference on Singapore Math strategies with a focus on visualization. It includes 4 sample math tasks: 1) finding the area of a 4-sided polygon on a geoboard; 2) calculating leftover wire used to make a figure of 6 equilateral triangles; 3) dividing numbers by 3, 4, and 6; and 4) a word problem about Peter and Nancy exchanging coins to determine how many Peter originally had. The tasks are meant to illustrate ways to get students to visualize math concepts.
Sd02 challenge me



Sd02 challenge meJimmy Keng The document discusses strategies for differentiated instruction for advanced learners. It focuses on enrichment rather than acceleration. It provides 6 case studies as examples of anchor tasks that can challenge advanced learners in lessons, including problems about number sequences, geometry of rooms and chairs, properties of triangles, and finding sums of hexagon angles. The goal of the session is to explore general strategies for differentiating instruction to meet the needs of advanced students.
Ad
Recently uploaded (20)
ANTI-VIRAL DRUGS unit 3 Pharmacology 3.pptx



ANTI-VIRAL DRUGS unit 3 Pharmacology 3.pptxMayuri Chavan ANTI-VIRAL DRUGS unit 3 Pharmacology 3.pptx
How to Share Accounts Between Companies in Odoo 18



How to Share Accounts Between Companies in Odoo 18Celine George In this slide we’ll discuss on how to share Accounts between companies in odoo 18. Sharing accounts between companies in Odoo is a feature that can be beneficial in certain scenarios, particularly when dealing with Consolidated Financial Reporting, Shared Services, Intercompany Transactions etc.
Form View Attributes in Odoo 18 - Odoo Slides



Form View Attributes in Odoo 18 - Odoo SlidesCeline George Odoo is a versatile and powerful open-source business management software, allows users to customize their interfaces for an enhanced user experience. A key element of this customization is the utilization of Form View attributes.
How to Manage Upselling in Odoo 18 Sales



How to Manage Upselling in Odoo 18 SalesCeline George In this slide, we’ll discuss on how to manage upselling in Odoo 18 Sales module. Upselling in Odoo is a powerful sales technique that allows you to increase the average order value by suggesting additional or more premium products or services to your customers.
How to Create Kanban View in Odoo 18 - Odoo Slides



How to Create Kanban View in Odoo 18 - Odoo SlidesCeline George The Kanban view in Odoo is a visual interface that organizes records into cards across columns, representing different stages of a process. It is used to manage tasks, workflows, or any categorized data, allowing users to easily track progress by moving cards between stages.
U3 ANTITUBERCULAR DRUGS Pharmacology 3.pptx



U3 ANTITUBERCULAR DRUGS Pharmacology 3.pptxMayuri Chavan U3 ANTITUBERCULAR DRUGS Pharmacology 3.pptx
Chemotherapy of Malignancy -Anticancer.pptx



Chemotherapy of Malignancy -Anticancer.pptxMayuri Chavan Chemotherapy of Malignancy -Anticancer.pptx
antiquity of writing in ancient India- literary & archaeological evidence



antiquity of writing in ancient India- literary & archaeological evidencePrachiSontakke5 for the students of BA Sem
Botany Assignment Help Guide - Academic Excellence



Botany Assignment Help Guide - Academic Excellenceonline college homework help Struggling with your botany assignments? This comprehensive guide is designed to support college students in mastering key concepts of plant biology. Whether you're dealing with plant anatomy, physiology, ecology, or taxonomy, this guide offers helpful explanations, study tips, and insights into how assignment help services can make learning more effective and stress-free.
📌What's Inside:
• Introduction to Botany
• Core Topics covered
• Common Student Challenges
• Tips for Excelling in Botany Assignments
• Benefits of Tutoring and Academic Support
• Conclusion and Next Steps
Perfect for biology students looking for academic support, this guide is a useful resource for improving grades and building a strong understanding of botany.
WhatsApp:- +91-9878492406
Email:- [email protected]
Website:- https://onlinecollegehomeworkhelp.com/botany-homework-help
Myopathies (muscle disorders) for undergraduate



Myopathies (muscle disorders) for undergraduateMohamed Rizk Khodair herediatary myopthies
myotonia
inflammatory myopthies
2025 The Senior Landscape and SET plan preparations.pptx



2025 The Senior Landscape and SET plan preparations.pptxmansk2 2025 The Senior Landscape and SET plan preparations.pptx
Cultivation Practice of Turmeric in Nepal.pptx



Cultivation Practice of Turmeric in Nepal.pptxUmeshTimilsina1 Cultivation Practice of Turmeric in Nepal
How to Configure Public Holidays & Mandatory Days in Odoo 18



How to Configure Public Holidays & Mandatory Days in Odoo 18Celine George In this slide, we’ll explore the steps to set up and manage Public Holidays and Mandatory Days in Odoo 18 effectively. Managing Public Holidays and Mandatory Days is essential for maintaining an organized and compliant work schedule in any organization.
*"Sensing the World: Insect Sensory Systems"*



*"Sensing the World: Insect Sensory Systems"*Arshad Shaikh Insects' major sensory organs include compound eyes for vision, antennae for smell, taste, and touch, and ocelli for light detection, enabling navigation, food detection, and communication.
Transform tomorrow: Master benefits analysis with Gen AI today webinar, 30 A...



Transform tomorrow: Master benefits analysis with Gen AI today webinar, 30 A...Association for Project Management Transform tomorrow: Master benefits analysis with Gen AI today webinar
Wednesday 30 April 2025
Joint webinar from APM AI and Data Analytics Interest Network and APM Benefits and Value Interest Network
Presenter:
Rami Deen
Content description:
We stepped into the future of benefits modelling and benefits analysis with this webinar on Generative AI (Gen AI), presented on Wednesday 30 April. Designed for all roles responsible in value creation be they benefits managers, business analysts and transformation consultants. This session revealed how Gen AI can revolutionise the way you identify, quantify, model, and realised benefits from investments.
We started by discussing the key challenges in benefits analysis, such as inaccurate identification, ineffective quantification, poor modelling, and difficulties in realisation. Learnt how Gen AI can help mitigate these challenges, ensuring more robust and effective benefits analysis.
We explored current applications and future possibilities, providing attendees with practical insights and actionable recommendations from industry experts.
This webinar provided valuable insights and practical knowledge on leveraging Gen AI to enhance benefits analysis and modelling, staying ahead in the rapidly evolving field of business transformation.
spinal cord disorders (Myelopathies and radiculoapthies)



spinal cord disorders (Myelopathies and radiculoapthies)Mohamed Rizk Khodair Myelopathies
Radiculopathies
BÀI TẬP BỔ TRỢ TIẾNG ANH 9 THEO ĐƠN VỊ BÀI HỌC - GLOBAL SUCCESS - CẢ NĂM (TỪ...



BÀI TẬP BỔ TRỢ TIẾNG ANH 9 THEO ĐƠN VỊ BÀI HỌC - GLOBAL SUCCESS - CẢ NĂM (TỪ...Nguyen Thanh Tu Collection https://app.box.com/s/yfwqwcillls0z4p34958pr8nj0b4udyr
How to Manage Amounts in Local Currency in Odoo 18 Purchase



How to Manage Amounts in Local Currency in Odoo 18 PurchaseCeline George In this slide, we’ll discuss on how to manage amounts in local currency in Odoo 18 Purchase. Odoo 18 allows us to manage purchase orders and invoices in our local currency.
Transform tomorrow: Master benefits analysis with Gen AI today webinar, 30 A...



Transform tomorrow: Master benefits analysis with Gen AI today webinar, 30 A...Association for Project Management
BÀI TẬP BỔ TRỢ TIẾNG ANH 9 THEO ĐƠN VỊ BÀI HỌC - GLOBAL SUCCESS - CẢ NĂM (TỪ...



BÀI TẬP BỔ TRỢ TIẾNG ANH 9 THEO ĐƠN VỊ BÀI HỌC - GLOBAL SUCCESS - CẢ NĂM (TỪ...Nguyen Thanh Tu Collection
Bendermeer Primary School Seminar for Parents
- 1. PEM 121 Helping Children with Primary Mathematics with a focus on Primary 4 – 6 Dr Yeap Ban Har Marshall Cavendish Institute Singapore [email protected] Slides are available at www.facebook.com/MCISingapore Da Qiao Primary School, Singapore
- 2. Mathematics is “an excellent vehicle for the development and improvement of a person’s intellectual competence”. Ministry of Education, Singapore (2006) thinkingschools learningnation
- 3. Ministry of Education, Singapore (1991, 2000, 2006, 2012)
- 5. Reflection of the Shifts in the Test Questions When we compare the tests from the past with the present, we see that: • Questions from previous tests were simpler, one or two steps, or were heavily scaffolded. The new questions will requires multiple steps involving the interpretation of operations. • Questions from the past were heavy on pure fluency in isolation. The new questions require conceptual understanding and fluency in order to complete test questions. • Questions from past tests isolated the math. The new problems are in a real world problem context. • Questions of old relied more on the rote use of a standard algorithm for finding answers to problems. The new questions require students to do things like decompose numbers and/or shapes, apply properties of numbers, and with the information given in the problem reach an answer. Relying solely on algorithms will not be sufficient. Department of Education New York State (2013) 5
- 6. What is Happening Around the World?Bringing Up Children Who Are Ready for the Global, Technological World
- 7. Da Qiao Primary School, Singapore 17 – 3 = 17 – 8 = Primary 1 Singapore 7
- 8. Da Qiao Primary School, Singapore Primary 1 Singapore 8 Learning by Doing Learning by Interacting Learning by Exploring Gardner’s Theory of Intelligences Bruner’s Theory of Representations Dienes’ Theory of Learning Stages
- 9. St Edward School, Florida Grade 2 USA 9
- 10. St Edward School, Florida Grade 2 USA 10
- 11. Archipelschool De Tweemaster – Kameleon, The Netherlands Grade 5 The Netherlands 11
- 12. Archipelschool De Tweemaster – Kameleon, The Netherlands Grade 5 The Netherlands 12
- 13. Archipelschool De Tweemaster – Kameleon, The Netherlands Grade 5 The Netherlands 13
- 14. mathematics 14 Students in Advanced Benchmark can • apply their understanding and knowledge in a variety of relatively complex situations • and explain their reasoning.
- 15. King Solomon Academy, London Year 7 England 15
- 16. 16 The sum of the two numbers is 88. The greater number is 6 x (88 11) = 48. The other number is 5 x (88 11) = 40.
- 17. High School Attached to Tsukuba University, Japan Draw a polygon with no dots inside it. Investigate. A polygon has 4 dots on the perimeter. Find an expression for its area. Grade 9 Japan 17
- 18. What Do Children Learn in School Mathematics? And How You Can Coach Them
- 19. Students who have mastered the basic skills which include basic one-step and two-step problems are ready to handle at least the least demanding of the secondary courses. Jay Sam 34.7 kg 34.7 kg x 2 = (68 + 1.4) kg 34.7 kg x 2 = 69.4 kgSam’s mass is 69.4 kg. 19
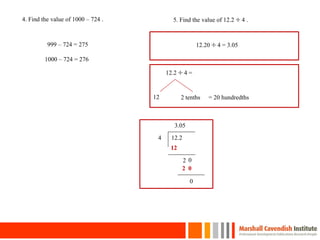
- 20. 4. Find the value of 1000 – 724 . 5. Find the value of 12.2 4 . 20 999 – 724 = 275 1000 – 724 = 276 12.20 4 = 3.05 12.2 4 = 12 2 tenths = 20 hundredths 12.24 12 2 0 2 0 0 3.05
- 21. What Are the Challenging Aspects of Mathematics? And How Children Develop Competencies to Handle Them
- 22. Problem 1 Mr Lim packed 940 books equally into 8 boxes. What was the least number of additional books he would need so that all the boxes contained the same number of books? Answer:__________ 22 940 8 = 117.5 4 118 × 8 = 944
- 23. Problem 1 Cup cakes are sold at 40 cents each. What is the greatest number of cup cakes that can be bought with $95? Answer:_____________ 23 $95 40 cents = 237.5 237
- 24. Problem 1 Cup cakes are sold at 40 cents each. What is the greatest number of cup cakes that can be bought with $95? Answer:_____________ 24 237
- 25. Problem 2 Mr Tan rented a car for 3 days. He was charged $155 per day and 60 cents for every km that he travelled. He paid $767.40. What was the total distance that he travelled for the 3 days? 25 $155 x 3 = $465 $767.40 - $465 = $302.40 $302.40 60 cents / km = 504 km He travelled 504 km.
- 26. Problem 2 Mr Tan rented a car for 3 days. He was charged $155 per day and 60 cents for every km that he travelled. He paid $767.40. What was the total distance that he travelled for the 3 days? 26 (767.40 - 155 x 3) 0.60 = 504 He travelled 504 km.
- 27. 27 (25 + 2) 3 = 9 9 + 1 = 10 10 x 8 + 25 = 105 105
- 28. 28 11m + 6 = 8(m + 1) + 25 3m = 27 m = 9 10511m + 6 = 99 + 6 = 105
- 29. 29 Number of Girls 11 sweets 8 sweets 105 2 11 + 6 16 + 25 3 22 + 6 24 + 25 4 33 + 6 32 + 25
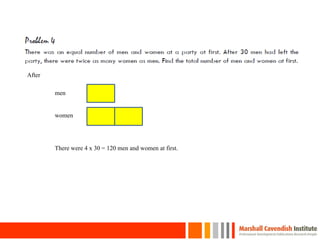
- 30. 30 men women There were 4 x 30 = 120 men and women at first. After
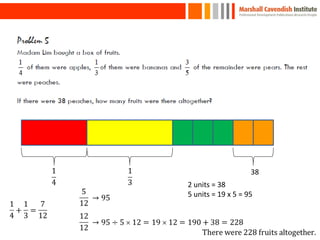
- 31. 31 2 fifths of the remainder were 38 3 fifths of the remainder were 19 x 3 = … There were 19 x 5 pears and peaches. 5 twelfths of the fruits = 19 x 5 fruits So, there were 19 x 12 fruits altogether. Answer: 228 fruits
- 32. 38 2 units = 38 5 units = 19 x 5 = 95 32
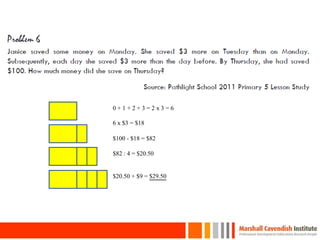
- 33. 33 0 + 1 + 2 + 3 = 2 x 3 = 6 6 x $3 = $18 $100 - $18 = $82 $82 : 4 = $20.50 $20.50 + $9 = $29.50
- 34. • Number Sense • Patterns • Visualization • Communication • Metacognition Five Core Competencies Try to do as you read the problems. Do not wait till the end of the question to try to do something. Try to draw when you do not get what the question is getting at. Diagrams such as models are very useful. Do more mental computation when practising Paper 1.
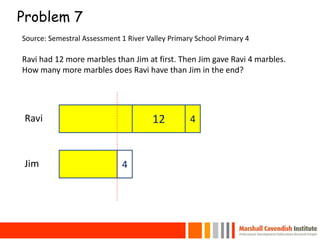
- 35. Problem 7 35 Source: Semestral Assessment 1 River Valley Primary School Primary 4 Ravi had 12 more marbles than Jim at first. Then Jim gave Ravi 4 marbles. How many more marbles does Ravi have than Jim in the end? 12Ravi Jim 4 4
- 36. Problem 8 36 Source: Semestral Assessment 1 Keming Primary School Primary 5 Daniel had only $2, $5 and $10 notes in his wallet. The ratio of the number of $2 notes to the number of the other notes was 5 : 3. The number of $5 notes was 3 times as many as that of $10 notes. If there were $460 in his wallet, how many $2 notes did he have? 12 parts 20 parts 9 parts 3 parts 20 parts x ($2) 9 parts x ($5) 3 parts x ($10) 115 units = $460 40 units = $160 He had 80 $2 notes.
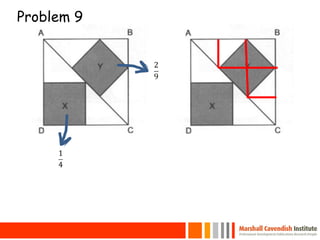
- 37. Problem 9 37
- 38. (a) 41 is under M (b) 101 is under S (c) 2011 is under T …. Really? How do you know? Problem 10
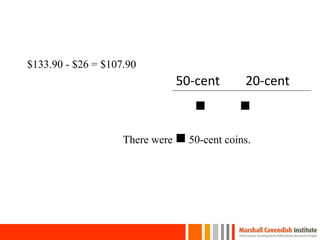
- 39. Problem 11 Weiyang started a savings plan by putting 2 coins in a money box every day. Each coin was either a 20-cent or 50-cent coin. His mother also puts in a $1 coin in the box every 7 days. The total value of the coins after 182 days was $133.90. (a) How many coins were there altogether? (b) How many of the coins were 50-cent coins? 182 7 = … 2 x 182 + 26 = …
- 40. $133.90 - $26 = $107.90 There were 50-cent coins. 50-cent 20-cent
- 41. Suppose each day he put in one 20-cent and one 50-cent coins, the total is $127.40 But he only put in $107.90 .. to reduce this by $19.50, exchange 50-cent for 20-cent coins $19.50 $0.30 = 65 There were 182 – 65 = 117 fifty-cent coins.








