The Map is Not the Territory

It's really no secret I love tape. I should have bought stock in it long ago.  In my mind, tape is the ultimate open-ended, the world is your oyster, creative, hands-on learning and making supply.  I mean, just look at how versatile it is:

You can change an environment in an instant!  Need kids to be able to visualize diagonals while creating foot-based percussive patterns? Voila!



Are you six and want to visualize a trajectory to the moon?  Tape's for you!



Even clear packing tape can be harnessed in the pursuit of art making and invention:



Check out the endless ways tape can be employed in the interest of math, art, kinesthetic exploration, invention and education -- and then consider submitting some examples of your own!  The Tape Chronicles are housed over at the Math in Your Feet website.  Hope to hear from you!

Saturdays we walk to the farmers market.  It's two miles from our house.  We visit our favorite booths, visit with our favorite market friends and, every summer, make new friends too.  And, we go to see the kittens the animal shelter brings every week.

The kitties (and the assorted teenagers who hang out with them) are all housed inside the lobby of City Hall.  Today, the two grey kittens were darling and the young woman who my almost eight-year-old has dubbed "the dramatic teenager' was teaching my kid how to sing.

I found myself with some (incredibly rare) time to think my own thoughts.  My phone got boring quite quickly, but I noticed some art on the walls so I wandered over to take a look.  Turns out they were prints of rubbings made from sewer covers, one of my very favorite places to find fascinating visual math.

The first picture has a fun math-y overlay in the reflection...see it?




So much to look at and take in.

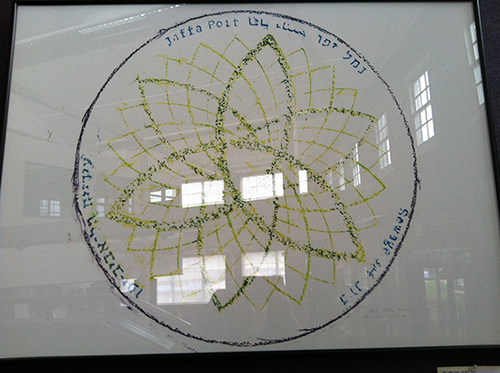
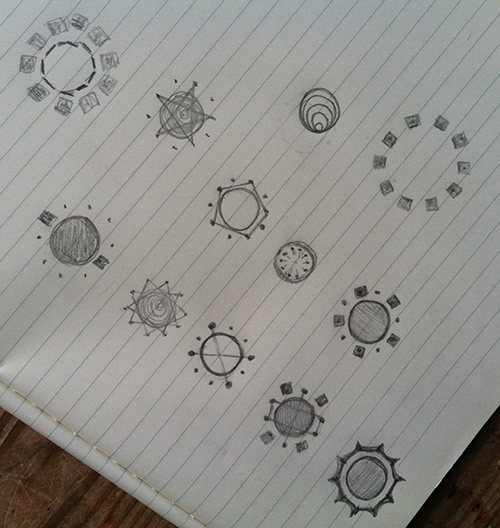
I went and found a comfy bench and pulled out my notebook and a not-very-sharp pencil.  From across the lobby I could see my favorite image, the one with squares in a circle.  Satisfied with sitting I squinted across the space and tried to figure out how many squares made the inner circle.

And then I started doodling.


At first I tried to freehand some squares in a circle.  I thought about angles.  I thought about how I didn't want there to be eight, because that's too easy.  So, I picked ten.  I thought about what it meant to freehand a circle.  I thought about how I liked that using an un-sharp pencil made me really think about precision.  I thought about how I was getting better at spacing ten dots around each circle.  I asked myself, how many different ways can I connect these dots to make something interesting?

On the walk home, things literally came full circle when we saw this:


I love Saturdays.

As the mother of a narrative-driven first grader, I have become a huge fan of the math storybooks out there.  I wish there had been more of them when I was a kid.  My daughter and I love books written by or supported by Marilyn Burns, like One Hungry Cat by Joanne Rocklin (it's about division).  I also like Loreen Leedy's books, on all sorts of math topics, and the Math Start series as well.  Our favorites are probably all the books in the Sir Circumference Series. 

On the other hand, we've really enjoyed learning math by finding it wherever we are and in whatever we're doing.  Since about February we've been paying attention to our physical world and finding as many different examples of geometry in our lives as we can.  It's quite stunning how beautiful and full of math even a city sidewalk can be if you have your math glasses on. 

Back in May I wanted to start looking for spirals but only found two examples.  One in a garden and one in our local playground.  Long story short, at some point my daughter picked up on the spiral thing and started pointing them out, only to have me say, "No, those are actually concentric circles," which then lead to a few days of clarification about what a spiral is and isn't. 

Now she sees them everywhere!  We're a team, her and I.  It's really fun that things we have taken for granted all our lives suddenly have a new dimension. Which is why, I think, that a recent return to reading familiar picture books from our home library made me notice math in otherwise non-mathy books.  Here are some examples.  Maybe you have more?

In Ezra Jack Keats' The Snowy Day, cut paper illustrations show math from the very first pages.  In addition to great spatial vocabulary (up and down the hills, tracks in the snow, on top of, snowballs flying over the boy's head) patterns abound. Check out this wallpaper -- I love how the pattern units are so different from each other, and yet the overall pattern is so regular:























Parallel lines made by sticks and feet and gates:


























I love this grid pattern in the mother's dress, and it's not just a color pattern.  If you look closely there's another attribute of shading (solid and striped):


























This background is a great example of 'scattered' like in a scatter plot.  Which section has more dots, and which has less?  How do you know:



















In nature, every snowflake has the same structure yet each one is different from every other snowflake.  That's not exactly the case here.  How many different kinds of snowflakes can you find?  How are they different and how are they the same?

























Caps for Sale by Esphyr Slobodkina is another classic picture book and also full of pattern and sequencing.  In my summer work recently, and also from years of work in my residencies, it's clear to me that children don't have enough experience identifying patterns and sequences in different contexts. 

"First he had on his own checked cap, then a bunch of gray caps, then a bunch of brown caps..."  

























I imagine that none of this is new news, but with my new math eyes if I ever have the opportunity to read this book to a three or four year old again I will probably pause the story after the monkeys throw down all the caps and see if the child can tell me the order the caps should be in when the peddler puts them back on.  And then have fun dressing up with our own hats!

One final book, for now, is our beloved Llama Llama Red Pajama book by Anna Dewdney.  I still remember leaving early one morning for work when my kid was 22 months old and having her look up at me reprovingly and quote the line:  "Llama llama red pajama, waiting waiting for her mama..."  Sniff. 

Anyhow, when you first open up the cover you see an incredible variety of painted squares that show up again on little llama's quilt.  Look at all those colors!  And the patterns?  Some are circles some are lines, but there are differences.  Can you tell me what they are? 
















My eye was also drawn to the shapes in the doors, windows and railings within the illustrations.  This story is so engaging that you may not want to interrupt the rhythmic, rhyming flow to discuss this stuff, but...if you're going to make comments about the wonderful illustrations anyhow, a few math themed comments like "Oh, look here!  I just noticed..." would fit in seamlessly.







































I think that any time a teacher or parent can share a discovery in the moment with a child or a group of children that there's potential for learning and understanding that might not be available otherwise.  I'm not saying that this approach is better than other approaches to teaching and learning out there, not at all.  But, I think that being in 'discovery mode' is a crucial piece of the learning puzzle.   My own program Math in Your Feet is one example of structured 'discovery' that includes a variety of approaches to learning (discussion, teacher led, student led, observation, writing, directing, group work, individual work, etc.) 

So, now I'm curious what other books are out there that have this kind of 'hidden' math?  I just thought of one more book: My daughter listened to the novel Half Magic on CD back in the fall.  The kids find a charm that gives them half their wish, and they quickly learn to wish for twice as much as they want.  It's fabulous.

What other kinds of books have this kind of hidden math?  I'd love to hear your ideas!

p.s.  If it's not already clear, I am not financially involved in any of the books I've mentioned or trying to make money with the use of these images.  This blog is about sharing ideas and thoughts only.

It's sort of a convoluted story* about how I came upon this cartoon but when I first read it I burst out laughing and thought how perfect it was for what I'm doing these days. (Meaning, trying to teach math to my child who creates a back story or narrative for pretty much any any endeavor.)  So, I thought I'd share.  The image came from Lynda Barry's blog The Near Sighted Monkey.



*The long story, if you're interested, is that Lynda Barry went to my alma mater The Evergreen State College in Olympia, WA.  She is a contemporary of Matt Groening (The Simpsons creator and Evergreen grad) and is, by all accounts, an incredible writing teacher.  I have been working up the courage to contact her to see if she might be interested in writing  for ALT/space, the online project of the Teaching Artist Journal (which I edit).  While I was thinking about all this I found her blog and saw that she would be teaching at a summer writing institute in my town.  That was a weird coincidence.  I heard through the grapevine that she is as wonderful as everyone says.  Although I have not yet worked up enough courage to get in touch, I have really been enjoying her blog!